Heated Specimen with Free Boundaries

Note

The project file for this example may be viewed/run in PFC. The data files used are shown at the end of this example.

A temperature change, \(\Delta T\), is applied to a cubic specimen with unconstrained boundaries. The thermally induced strains are measured and compared with analytical values for an isotropic elastic continuum.

Analytical Values

The stress-strain relation with thermally induced strain is given by Equation (1) ([Timoshenko1970b]):

(1)\[\begin{split}\epsilon_x &= {1 \over E} {\big[ \sigma_x - \nu (\sigma_y+\sigma_z) \big]} + \alpha_t \Delta T \\ \epsilon_y &= {1 \over E} {\big[ \sigma_y - \nu (\sigma_z+\sigma_x) \big]} + \alpha_t \Delta T \\ \epsilon_z &= {1 \over E} {\big[ \sigma_z - \nu (\sigma_x+\sigma_y) \big]} + \alpha_t \Delta T\end{split}\]

where \(E\) and \(\nu\) are the Young’s modulus and the Poisson’s ratio, respectively. Also, \(\alpha_t\) is the coefficient of linear thermal expansion.

The analytical values for the thermally induced strains with free boundaries are given by Equation (2):

(2)\[\epsilon_x = \epsilon_y = \epsilon_z = \alpha_t \Delta T\]

Model and Results

The file “free_expansion.dat” is used for this example. A 2 × 2 × 2 m specimen is created. It comprises approximately 4000 balls with uniform size distribution (radius range from 0.05 to 0.08 m), with a porosity of 0.36. Once the specimen is generated, contact bonds are installed at ball-ball contacts, the surrounding walls are deleted, and the specimen is brought to equilibrium.

The strains are measured using a measurement sphere with a radius of 0.95 m, centered within the specimen. In PFC, the particle thermal-expansion coefficient is the same as the coefficient of linear thermal expansion: \(\alpha_t\) = 3 × 10^-6/°C, in this case.

The expected strains for \(\Delta T\) = +100°C are

(3)\[\epsilon_x = \epsilon_y = \epsilon_z = 3.0 \times 10^{-4}\]

The expected strains for \(\Delta T\) = -100°C are

(4)\[\epsilon_x = \epsilon_y = \epsilon_z = -3.0 \times 10^{-4}\]

Figure 1 and Figure 2 show displacement vectors resulting from the positive and negative temperature change; they show an isotropic expansion and isotropic contraction, respectively. Figure 3 and Figure 4 show histories of strain recorded during the expansion and contraction phases respectively.

The result of the simulation is in accordance with the analytical solution.

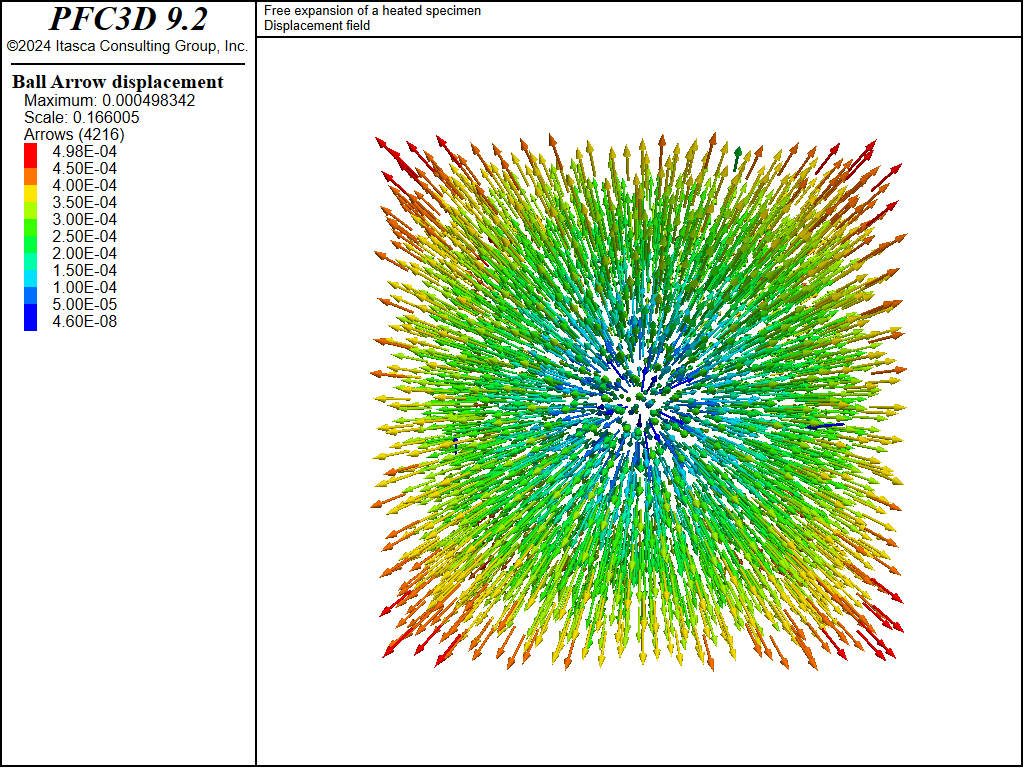
Figure 1: Displacement field at the end of thermal isotropic expansion.

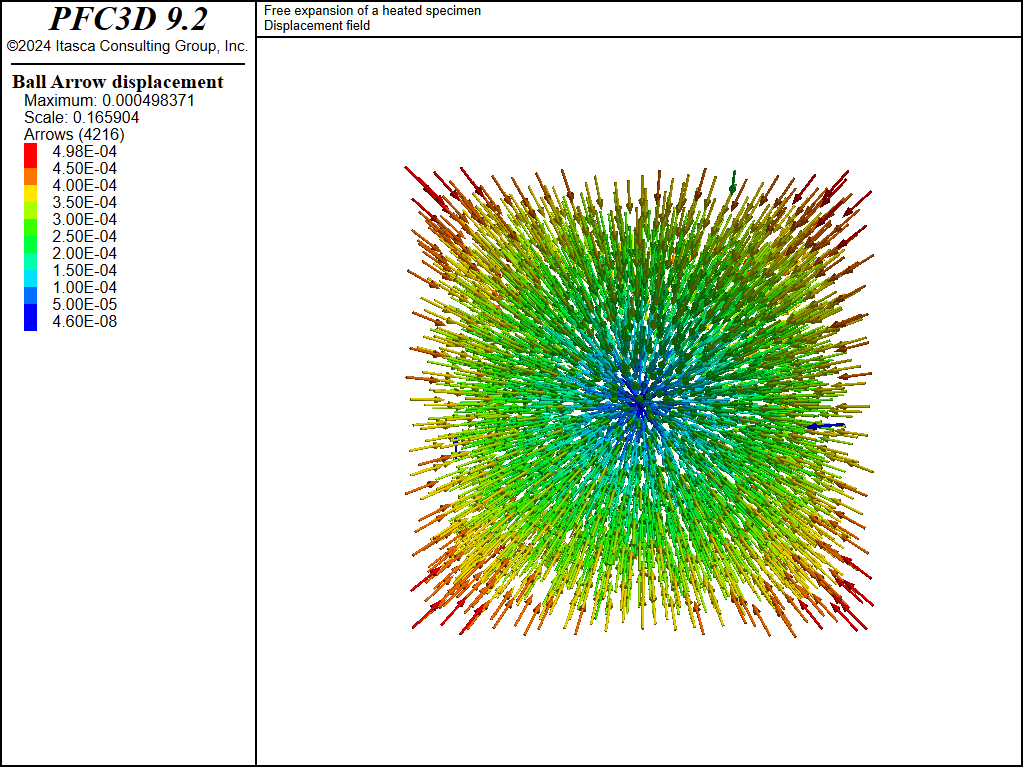
Figure 2: Displacement field at the end of thermal isotropic contraction.

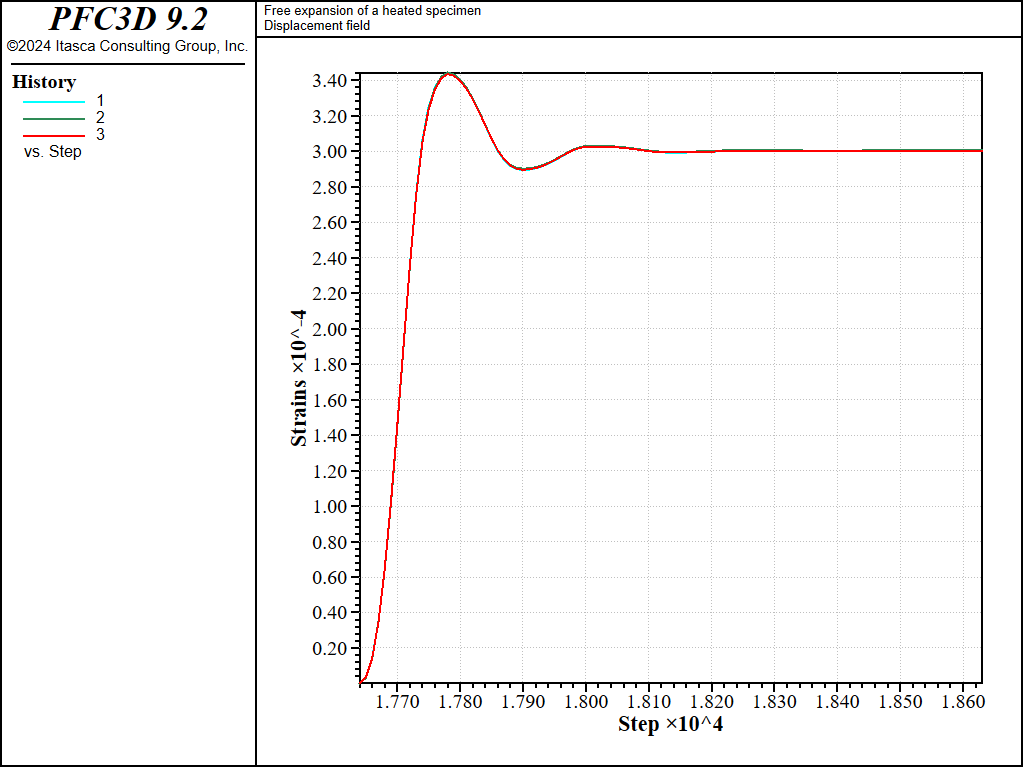
Figure 3: Strain histories at the end of thermal isotropic expansion.

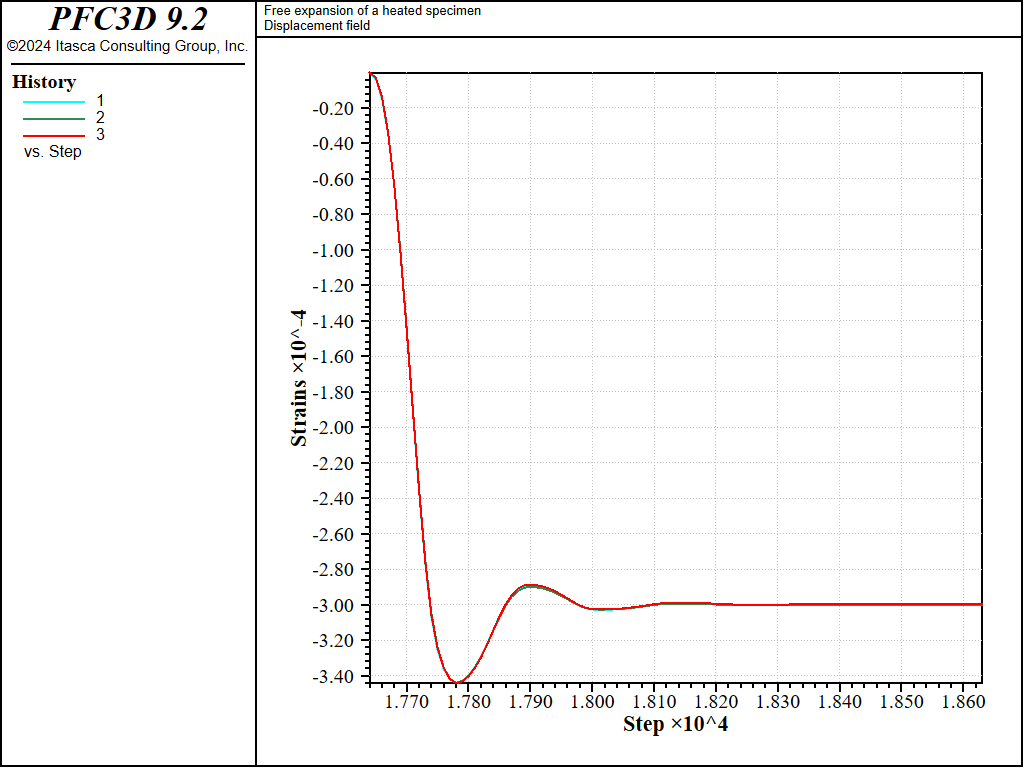
Figure 4: Strain histories at the end of thermal isotropic contraction.

References

[Timoshenko1970b]

Timoshenko, S. P., and J. N. Goodier. Theory of Elasticity, 3rd Ed. New York: McGraw-Hill, 1970.

Data Files

free_expansion.dat

; fname: free_expansion.dat (3D)
;
; Itasca Consulting Group, Inc.
; ========================================================================
; Thermal option verification problem:
;        Free expansion of a heated specimen
;
; ========================================================================
program log-file "free_expansion.log"
program log on
; ========================================================================
model new
model large-strain on
model title 'Free expansion of a heated specimen'

; build specimen

model domain extent -5 5
contact cmat default type ball-ball    ... *
              model linearcbond ...
              method deformability emod 3.81e8 kratio 1.0 proximity 0.005

contact cmat default type ball-facet   ...
              model linear     ...
              method deformability emod 3.81e8 kratio 1.0


wall generate box -1 1
model random 10001
ball distribute porosity 0.36     ...
                 radius 0.05 0.08 ...
                 box -1 1

ball attribute position multiply 0.95
ball attribute density 1500.0 damp 0.7
model cycle 2000 calm 100
model mechanical timestep scale
model solve ratio-average 1e-4

contact method bond gap 0.005
contact property cb_tenF 1e20 cb_shearF 1e20
wall delete
ball attribute displacement multiply 0.0 
model cycle 1000
model mechanical timestep automatic
model solve ratio-average 1e-6


; install measurement sphere and activate strain calculation

measure create id 1 radius 0.95

fish define ini_mstrains
  global mp = measure.find(1)
  global mstrains = matrix(3,3)
end
[ini_mstrains]

fish define accumulate_mstrains
  global msrate =  measure.strain.rate(mp)
  global mstrains = mstrains + msrate * global.timestep
  global xxmstrain = mstrains(1,1)
  global yymstrain = mstrains(2,2)  
  global zzmstrain = mstrains(3,3)  
end

fish callback add accumulate_mstrains 11.0
fish history xxmstrain
fish history yymstrain
fish history zzmstrain

model save "initial"

;---------------- case a: apply +100 deg C ----------------------------
model configure thermal
ball thermal attribute expansion 3.0e-6
ball thermal attribute specific-heat 1.0e3
ball thermal attribute temperature 0.0
ball thermal attribute temperature-increment=100.0
model cycle 1
model thermal off mechanical on
ball attribute displacement multiply 0.0
model cycle 1000
model solve ratio-average 1e-6
model save "final1"

;---------------- case b: apply -100 deg C ----------------------------
model restore "initial"
model configure thermal
ball thermal attribute expansion 3.0e-6
ball thermal attribute specific-heat 1.0e3
ball thermal attribute temperature 0.0
ball thermal attribute temperature-increment=-100.0
model thermal on mechanical on
model cycle 1
model thermal off mechanical on
ball attribute displacement multiply 0.0
model cycle 1000
model solve ratio-average 1e-6
model save "final2"

program log off
program return
; ========================================================================
; eof: free_expansion.dat (3D)